14.4:
Calculating the Equilibrium Constant
25,679 Views
•
•
The equilibrium constant for a reaction is calculated from the equilibrium concentrations (or pressures) of its reactants and products. If these concentrations are known, the calculation simply involves their substitution into the Kc expression.
For example, gaseous nitrogen dioxide forms dinitrogen tetroxide according to this equation:
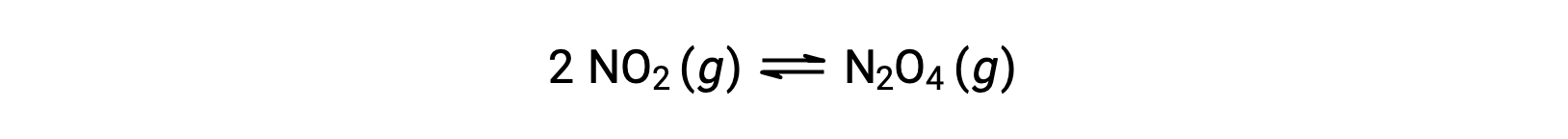
When 0.10 mol NO2 is added to a 1.0-L flask at 25 °C, the concentration changes so that at equilibrium, [NO2] = 0.016 M and [N2O4] = 0.042 M. The value of the equilibrium constant for the reaction can be calculated as follows:
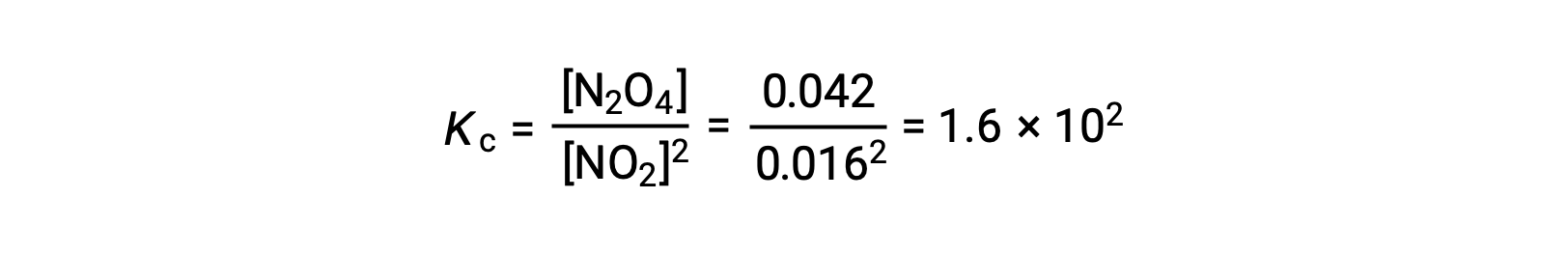
A slightly more challenging example is provided next, in which the reaction stoichiometry is used to derive equilibrium concentrations from the information provided. The basic strategy of this computation is helpful for many types of equilibrium computations and relies on the use of terms for the reactant and product concentrations initially present, for how they change as the reaction proceeds, and for what they are when the system reaches equilibrium. The acronym ICE is commonly used to refer to this mathematical approach, and the concentration terms are usually gathered in a tabular format called an ICE table.
Calculation of an Equilibrium Constant
Iodine molecules react reversibly with iodide ions to produce triiodide ions.
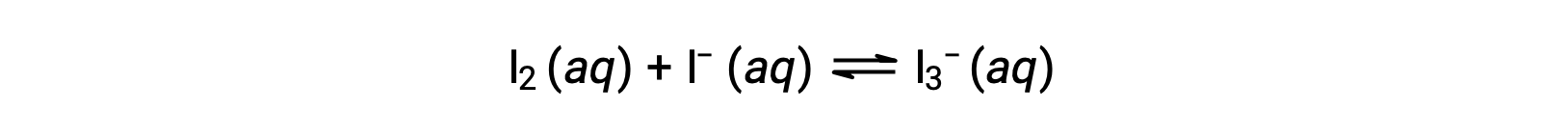
If a solution with the concentrations of I2 and I− both equal to 1.000 × 10−3 M before reaction gives an equilibrium concentration of I2 of 6.61 × 10−4 M, what is the equilibrium constant for the reaction?
To calculate the equilibrium constants, equilibrium concentrations are needed for all the reactants and products:
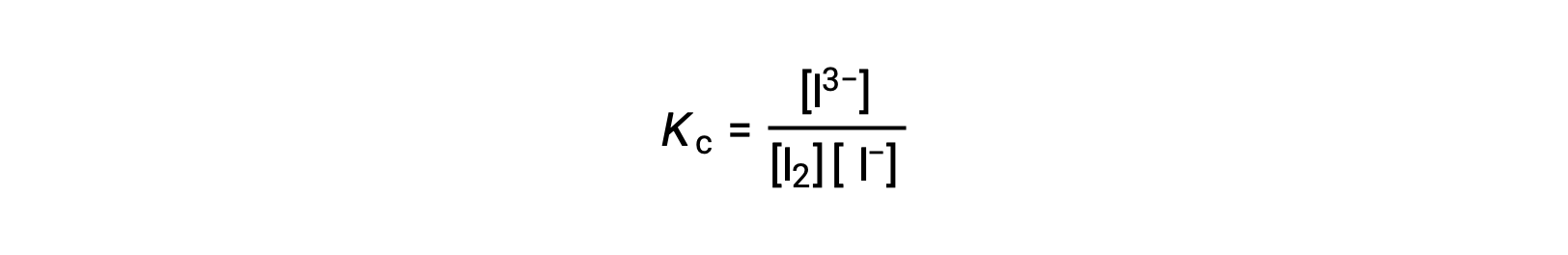
The initial concentrations of the reactants and the equilibrium concentration of the product are provided. This information can be used to derive terms for the equilibrium concentrations of the reactants, presenting all the information in an ICE table.
| I2 (aq) | I− (aq) | I3− (aq) | |
| Initial Concentration (M) | 1.000 × 10−3 | 1.000 × 10−3 | 0 |
| Change (M) | −x | −x | +x |
| Equilibrium Concentration (M) | 1.000 × 10−3 − x | 1.000 × 10-3 − x | x |
At equilibrium the concentration of I2 is 6.61 × 10−4 M so that
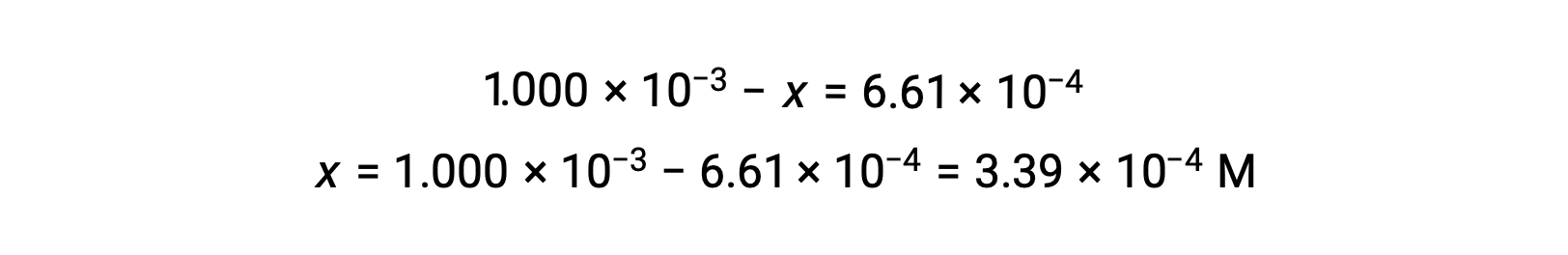
The ICE table may now be updated with numerical values for all its concentrations:
| I2 (aq) | I− (aq) | I3− (aq) | |
| Initial Concentration (M) | 1.000 × 10−3 | 1.000 × 10−3 | 0 |
| Change (M) | −3.39 × 10−4 | −3.39 × 10−4 | +3.39 × 10-4 |
| Equilibrium Concentration (M) | 6.61 × 10−4 | 6.61 × 10−4 | 3.39 × 10−4 |
Finally, the equilibrium concentrations can be substituted into the Kc expression and solved:
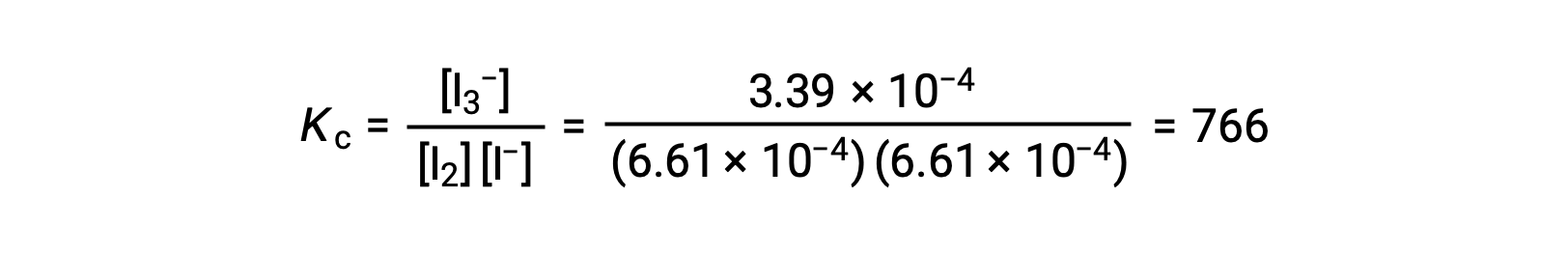
This text has been adapted from Openstax, Chemistry 2e, Section 13.4 Equilibrium Calculations.
