Quantifying the Relative Thickness of Conductive Ferromagnetic Materials Using Detector Coil-Based Pulsed Eddy Current Sensors

Nalika Ulapane; Karthick Thiyagarajan; David Hunt; Jaime Valls Miro

doi:10.3791/59618

JoVE Journal > Engineering

Ingeniería

Quantifying the Relative Thickness of Conductive Ferromagnetic Materials Using Detector Coil-Based Pulsed Eddy Current Sensors

Published: January 16, 2020

doi:

10.3791/59618

Nalika Ulapane¹, Karthick Thiyagarajan², David Hunt², Jaime Valls Miro²

¹Melbourne School of Engineering,University of Melbourne, ²Center for Autonomous Systems,University of Technology Sydney

Summary

Here, we present a protocol to quantify the relative thickness (i.e., thickness as a percentage with respect to a reference) of conductive ferromagnetic materials using detector coil-based pulsed eddy current sensors, while overcoming the calibration requirement.

Abstract

Thickness quantification of conductive ferromagnetic materials by means of non-destructive evaluation (NDE) is a crucial component of structural health monitoring of infrastructure, especially for assessing the condition of large diameter conductive ferromagnetic pipes found in the energy, water, oil, and gas sectors. Pulsed eddy current (PEC) sensing, especially detector coil-based PEC sensor architecture, has established itself over the years as an effective means for serving this purpose. Approaches for designing PEC sensors as well as processing signals have been presented in previous works. In recent years, the use of the decay rate of the detector coil-based time domain PEC signal for the purpose of thickness quantification has been studied. Such works have established that the decay rate-based method holds generality to the detector coil-based sensor architecture, with a degree of immunity to factors such as sensor shape and size, number of coil turns, and excitation current. Moreover, this method has shown its effectiveness in NDE of large pipes made of grey cast iron. Following such literature, the focus of this work is explicitly PEC sensor detector coil voltage decay rate-based conductive ferromagnetic material thickness quantification. However, the challenge faced by this method is the difficulty of calibration, especially when it comes to applications such as in situ pipe condition assessment since measuring electrical and magnetic properties of certain pipe materials or obtaining calibration samples is difficult in practice. Motivated by that challenge, in contrast to estimating actual thickness as done by some previous works, this work presents a protocol for using the decay rate-based method to quantify relative thickness (i.e., thickness of a particular location with respect to a maximum thickness), without the requirement for calibration.

Introduction

The pulsed eddy current (PEC) sensing technique is perhaps the most versatile member of the family of eddy current (EC) non-destructive evaluation (NDE) techniques and has many applications in detection and quantification of defects, and the geometry of metals and metallic structures¹. Thickness quantification of conductive ferromagnetic wall-like structures, having wall thicknesses of no more than a few millimeters to a few tens of millimeters, is a high demand engineering service in the field of structural health monitoring of infrastructure. Critical infrastructure made of ferromagnetic alloys that require this service are commonly available in the energy, water, oil, and gas industries. While PEC sensors can be designed following several architectures, the detector coil-based architecture was determined to be the most effective and commonly used in condition assessment of ferromagnetic materials²^,³^,⁴^,⁵. Therefore, it is the detector coil-based PEC sensor architecture that sets the foundation to the problem of thickness quantification of conductive ferromagnetic materials.

The detector coil-based PEC sensor architecture is typically comprised of two concentrically wound, air cored, conductive coils²^,³^,⁴^,⁵^,⁶ (typically copper coils). It is quite common to wind these coils to be circular in shape²^,³^,⁴^,⁵^,⁶, but occasionally, rectangular shaped coils⁶ have been used. From the two coils in the sensor, one behaves as an exciter coil while the other acts as the detector coil. In a PEC sensor, the exciter coil is excited by a voltage pulse – something that can be characterized as a Heaviside step function in principle. This pulsed excitation generates a transient magnetic field (called the primary field) around the sensor. When the sensor is placed adjacent to a conductive test piece (e.g., a conductive ferromagnetic wall-like structure), this transient magnetic field induces time varying eddy currents in the test piece. These eddy currents generate a secondary magnetic field (called the secondary field) that opposes the primary field. In response to the resultant effect of the primary and secondary fields, a transient voltage is induced in the detector coil – which becomes the time domain PEC signal of interest for this work.

The PEC sensor detector coil voltage decay rate (denoted as β) has been reported⁶^,⁷^,⁸ to show the proportionality β 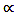μσd², when a signal is acquired placing a PEC sensor above a conductive ferromagnetic layer of magnetic permeability μ, electrical conductivity σ, and thickness d. Although this decay rate signal feature has considerable immunity to parameters such as sensor size, sensor shape, and lift-off⁶^,⁷^,⁸, which makes the decay rate highly desirable for challenging NDE scenarios such as in situ pipe condition assessment⁹^,¹⁰^,¹¹, this feature must be calibrated (i.e., μ, σ of the material being inspected be estimated) to enable thickness (i.e., d) quantification. To enable conventional methods of decay rate-based thickness quantification⁶^,⁸, this calibration must be done by extracting calibration samples⁶^,⁸ or by involving eddy current-based material property characterization methods¹²^,¹³. Alternatively, the complexity of calibration can be avoided by representing thickness in the form of relative thickness. Suppose an NDE exercise is carried out and β values are extracted from signals, then, the β value qualitatively representative of the maximum thickness point in the test piece is considered as a reference (i.e., β_ref 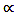μσd_max²); then, the thickness of any other location can be represented as a percentage of the maximum thickness in the form 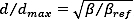, presenting a relative thickness as the output, which is still useful qualitative information as an NDE output that also carries the simplicity of not having to calibrate for μ, σ. The protocol presented herein describes the steps to be followed to accomplish this.

Since the decay rate β shows generality to the detector coil-based PEC sensor architecture while showing immunity to parameters of the sensor design as well as lift-off⁶^,⁷^,⁸^,¹⁴, practitioners may use any detector coil-based PEC sensing system of their choice on a suitable conductive ferromagnetic material to perform relative thickness quantification following the protocol here. A PEC sensor design example for a conductive ferromagnetic material is available for interested readers¹⁵. The signals and results presented in this work were acquired using the PEC system developed by University of Technology Sydney⁶^,⁸. The conductive ferromagnetic material used for representative results acquired by the PEC system is grey cast iron extracted from a pipe test-bed⁹^,¹⁰^,¹¹ in Sydney Australia.

It should be noted that the methods, results, and discussions presented in this publication explicitly focus on the use of the detector coil-based PEC sensor architecture's time domain signal's decay rate for thickness quantification of conductive ferromagnetic materials. The publication does not include a broader discussion on general conventions of PEC sensing principles and sensor configurations. Other published work¹⁶^,¹⁷^,¹⁸ can be useful for readers to gain more insight about PEC sensor configurations other than the detector coil-based sensor architecture.

Protocol

1. Extracting the decay rate β from an available detector coil-based PEC signal

Express an available experimentally captured PEC signal (i.e., a time domain detector coil voltage (denoted as V(t))) in the logarithmic form of ln[V(t)]. A typical PEC signal expressed in the form of ln[V(t)] is shown in Figure 1.
Find a linear region in the form of such that the signal satisfies the condition 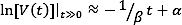where . As per the signal in Figure 1, 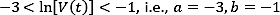happens to be a satisfactory and practicable linear region.
As illustrated in Figure 2, fit the straight-line model to the experimental signal data within the identified linear region and estimate the value of β.

2. Quantification of relative thickness

Suppose there are multiple signals (Figure 3) acquired from an NDE task performed on a conductive ferromagnetic test piece having varying thickness. First, identify a linear region common to all signals and extract β values. As per the signals in Figure 3, 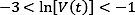seems to be an adequate and practicable linear region.
Select the maximum β value and label it as β_ref since the maximum β value should in principle correspond to the maximum thickness according to the β 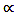μσd² proportionality⁶^,⁷^,⁸.
Express relative thickness percentage in the form 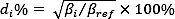, where the index 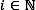corresponds to the 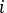^th measurement.

3. PEC_Signal_Processor installation

Locate the file PEC_Signal_Processor.exe. Double click the file and allow to execute.
When the interface below appears, click Next. When the interface pops up, specify the file location for installation, and tick the checkbox Add a shortcut to the desktop to add the software icon to the desktop. Then click Next.
Specify the installation location for the required Runtime Environment, then click Next. If the required Runtime Environment is already installed, just click Next.
Read, and agree to prompted license terms and conditions. Then click Install.
Click Finish when installation is complete. The desktop icon will appear.

4. Preparation of signals

Ensure the PEC sensor outputs [raw signals, i.e., V(t)] are arranged as a table.
Copy the table containing signals to the desktop (or to a folder contained within the parent directory where the application is installed). For convenience, the desktop is recommended.

5. Executing the application

Double click the desktop icon to run the application. The interface will open.
Load signals by clicking on the Load Signals tab and select the file containing the signals in order to import the signals to the software interface.
Wait until the number of signals which is contained within the table containing raw signals appear in front of Number of Signals =.
Click on Plot Signals and observe the signals plotted in logarithmic scale.
Click on the Zoom tab and adjust the plot window for the linear region to be clearly visible.
After observation, decide on reasonable lower and upper margins for the linear region and enter the values in the editable text spaces.
Click on Plot Margins and wait for the margins to be plotted in green.
Click on Extract Features and observe how straight-line segments are plotted in red.
Click on Calculate Relative Thickness and observe how a histogram of calculated relative thickness values is plotted.
Click on Save Relative Thickness to save the calculated relative thickness values. Provide a filename and click OK.
Confirm filename by clicking OK again to confirm the filename. The relative thickness values will be saved as a table on the desktop.

Representative Results

Representative results within this section have been generated using the PEC signals provided as supplementary material with reference⁸; as mentioned above, the signals have been captured on grey cast iron samples extracted from the pipe test bed in Sydney Australia, whose location and vintage details are provided in references⁹^,¹⁰^,¹¹.

Figure 1 shows the typical shape of a time domain signal (expressed in the logarithmic form) captured from a detector coil-based PEC sensor while Figure 2 shows an indicative linear region of the logarithmic signal as t >> 0 from which the decay rate feature β is extracted. Multiple PEC signals are shown in Figure 3; a signal showing an indicatively maximum β value is included. Such a maximum β value can be used as the reference β values to quantify relative thickness using the equation .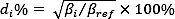

Table 1 shows some extracted β values along with relative thickness values that have been quantified using them. The results in Table 1 have been produced for the 14 mm lift-off case presented in Figure 20 of reference⁸; the corresponding raw PEC signals captured on grey cast iron are available in the supplementary material provided with reference⁸. The first column of Table 1 provides the actual thickness (in millimeters) of the grey cast iron test pieces on which the PEC signals were captured while the second column contains the corresponding β values. The maximum β value (i.e., β = 0.010078491) is considered as the reference β value (i.e., β_ref). Provided in the third column are the corresponding relative thickness values resulting when quantified as 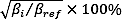and the last column lists the corresponding actual relative thickness values calculated using the actual thickness values listed in the first column. A plot of the actual relative thickness values against the relative thickness values calculated from PEC signals (i.e., calculated using β values) is shown in Figure 4. A correlation of over 99% between estimates and reality observed on this data set indicates the effectiveness of the relative thickness quantification method.

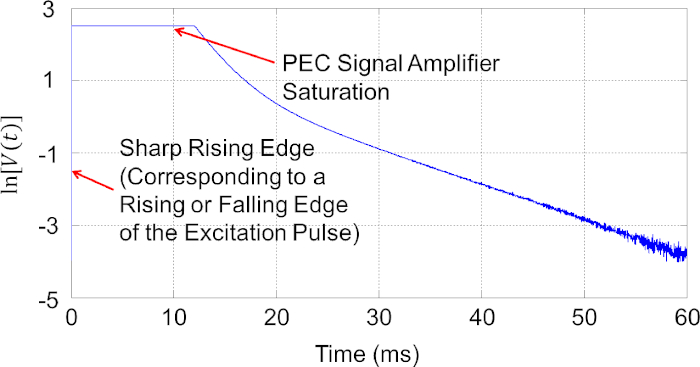
Figure 1: Typical shape of a PEC signal, i.e., induced detector coil voltage (i.e., V(t)) expressed in the form of ln[V(t)]. Please click here to view a larger version of this figure.

Figure 2: A representative linier region of a detector coil-based PEC signal expressed in the form ln[V(t)], as t >> 0, from where the decay rate feature (i.e., β) should be extracted. Please click here to view a larger version of this figure.

Figure 3: Multiple PEC signals resulting from an NDE exercise (performed on grey cast iron) displaying a signal indicatively showing a maximum β value, which should in principle qualitatively represent a maximum thickness according to the proportionality⁶^,¹⁵: β 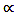μσd². Please click here to view a larger version of this figure.

Figure 4: Correlation between Relative Thickness % quantified from actual thickness and Relative Thickness % quantified from β (based on measurements performed on grey cast iron). Please click here to view a larger version of this figure.

Actual Thickness of Test Piece (mm)	β Value Extracted from PEC Signal	Relative Thickness % Quantified from β	Relative Thickness % Quantified from Actual Thickness
3	0.000228395	15.05379428	15
5	0.000538137	23.10728068	25
7	0.001018941	31.79631773	35
9	0.001701758	41.09141494	45
11	0.003091023	55.38005157	55
15	0.005853939	76.2125254	75
20	0.010078491	100	100

Table 1: Some extracted β values along with quantified relative thickness of grey cast iron.

Discussion

A protocol to quantify the relative thickness (i.e., thickness as a percentage with respect to a reference) of conductive ferromagnetic materials using detector coil-based PEC sensors was presented. The main advantage of this method is the ability to overcome the calibration requirement (i.e., overcome the need to measure or estimate the magnetic permeability and electrical conductivity of the material being inspected to enable thickness quantification). The protocol involves logarithmic representation of the time domain PEC signal, identification of a linear region at the signal's later stages, fitting a straight-line to the linear region and extracting the decay rate (i.e., β), and quantifying the relative thickness with respect to a reference through the equation 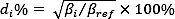, which cancels out the material properties (i.e., μσ) and produces a relative thickness due to the β 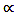μσd² proportionality. Experimental results (Table 1 and Figure 4) show the effectiveness of the relative thickness quantification method from the protocol in this work.

Ensure the guidelines in Section 4 are followed when preparing data for import to the software interface. To avoid a heavy burden on computation and graphics hardware, arrange signals into multiple tables so that data files of smaller size can be processed separately. It is not easy to impose actual restrictions on the size of data files as that depends on the computation power available to users. A few trial and error tests are recommended to identify any file size restrictions that may apply according to computation power available to users. In terms of modifications, users may be able to program their own software packages with multiple, or any PEC signal processing algorithms of their choice, using any computation platform of their choice. A review of recently published PEC signal processing algorithms for thickness quantification of conductive ferromagnetic materials is available¹⁹.

A crucial factor that will affect the accuracy of the estimated relative thickness values is the adequacy of the excitation strength. Reference⁸ has reported how too little excitation strength can limit the depth of penetration reducing sensitivity to high thickness values while too much excitation strength can limit the sensitivity to low thickness values. This issue with the excitation strength means that if a particular thickness range of a particular material is assessed with an inadequate excitation strength, although the resulting decay rate might still be sensitive to thickness, it may be inaccurate in value to universally follow the principle β 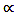μσd², which may eventually result in erroneous relative thickness values quantified as 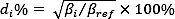. This limitation needs to be taken note of by practitioners in situations where strict precision in quantitative NDE results matter. However, this does not become an issue in situations where quantitative outputs are not essential and qualitative representation of the test piece condition suffices. Since defining a procedure to tune the excitation strength without the use of calibration samples having known thickness is nontrivial, a good practice to counter any ambiguity with the excitation strength will be to collect signals under a range of excitation strengths. Such approaches will generate rich data sets that form bases for some advanced postprocessing and may be useful for NDE tasks performed by robotic means¹⁰^,¹¹. Further, the electrical and magnetic properties of certain inhomogeneous conductive ferromagnetic materials, such as grey cast iron encountered in some aged critical water pipes, display a considerable variance⁸. This variance imposes that the properties be different within a cohort of pipes, or at times even within a single pipe from one location to another, making calibration all the more challenging. Such variation in material properties will also act as a source of error for the method prescribed in this work when NDE is performed on such inhomogeneous materials.

Recent work has demonstrated frequent and continued use of PEC sensing for condition assessment of critical pipes⁸^,⁹^,¹⁰^,¹¹^,²⁰^,²¹^,²². Such works do tend to produce large amounts of PEC data and would benefit from signal analysis protocols and frameworks similar to the one presented in this work. In parallel to work on critical pipes, there has been an increased interest in research on condition assessment of concrete sewers as well in recent years²³^,²⁴^,²⁵^,²⁶^,²⁷^,²⁸^,²⁹^,³⁰^,³¹. Along with such work, PEC sensing technique has found use in condition assessment of steel reinforced sewers as well³². PEC signal analysis protocols such as the one presented in this work can be useful for analysis of the vast amounts of PEC data produced as a result of such condition assessment-related work.

Critical steps of the method can be listed as: (1) arranging raw PEC signals [V(t)] as a table; (2) loading the raw signals to the software interface; (3) plotting the signals in logarithmic scale and visualizing (i.e., plotting signals in ln[V(t)] form); (4) visually inspecting the plotted signals and identifying a suitable linear range; (5) performing feature extraction; (6) performing relative thickness quantification; and (7) saving the results. Section 3 provides more detailed step-by-step guidelines to perform the tasks listed above.

Divulgaciones

The authors have nothing to disclose.

Acknowledgements

The authors would like to acknowledge the contributions made by Michael Behrens and Damith Abeywardana in designing and implementing several sensing hardware components. Research supervision roles played by Alen Alempijevic, Teresa Vidal-Calleja, Gamini Dissanayake, and Sarath Kodagoda, as well as contributions made by all persons and organizations who funded and partnered with the Critical Pipes Project, are also acknowledged.

Materials

A Detector Coil-based PEC Sensing System.	N/A	The representative results in this work were generated using the PEC system developed by University of Technology Sydney (UTS), Australia and published in works 6,8. This system may be accessible to readers via collaborating with UTS.
A suitable conductive ferromagnetic material of varying thickness.	N/A	The representative results in this work were generated by acquiring PEC measurements on grey cast iron test pieces extracted from a pipe test-bed located in Sydney Australia, whose location and vintage details are available in references 9-11. The pipe test-bed as well as the extracted calibration samples may be accessible to readers via collaborating with UTS.
A computation platform for PEC signal processing	MathWorks, Natick, MA, USA.	A computation platform in which the PEC signal processing algorithm can be coded and executed is required. In this publication, PEC signal processing was done using a software executable named "PEC_Signal_Processor", produced using MATLAB R2017b, Publisher: MathWorks, Natick, MA, USA.
An application that can produce a table containing raw PEC signals (e.g., Microsoft Office Excel).	Microsoft Corporation, One Microsoft Way, Redmond, Washington, USA.	Microsoft Office Excel (Office 16) was used for the work of this publication.

Referencias

García-Martín, J., Gómez-Gil, J., Vázquez-Sánchez, E. Non-destructive techniques based on eddy current testing. Sensors. 11 (3), 2525-2565 (2011).
Huang, C., Wu, X., Xu, Z., Kang, Y. Ferromagnetic material pulsed eddy current testing signal modeling by equivalent multiple-coil-coupling approach. Non-Destructive Testing and Evaluation International. 44 (2), 163-168 (2011).
Xu, Z., Wu, X., Li, J., Kang, Y. Assessment of wall thinning in insulated ferromagnetic pipes using the time-to-peak of differential pulsed eddy-current testing signals. Non-Destructive Testing and Evaluation International. 51, 24-29 (2012).
Huang, C., Wu, X. An improved ferromagnetic material pulsed eddy current testing signal processing method based on numerical cumulative integration. Non-Destructive Testing and Evaluation International. 69, 35-39 (2015).
Chen, X., Lei, Y. Electrical conductivity measurement of ferromagnetic metallic materials using pulsed eddy current method. Non-Destructive Testing and Evaluation International. 75, 33-38 (2015).
Ulapane, N., Alempijevic, A., Valls Miro, J., Vidal-Calleja, T. Non-destructive evaluation of ferromagnetic material thickness using Pulsed Eddy Current sensor detector coil voltage decay rate. Non-Destructive Testing and Evaluation International. 100, 108-114 (2018).
Ulapane, N., Nguyen, L., Valls Miro, J., Dissanayake, G. A Solution to the Inverse Pulsed Eddy Current Problem Enabling 3D Profiling. IEEE Conference on Industrial Electronics and Applications. , (2018).
Ulapane, N., Alempijevic, A., Vidal Calleja, T., Valls Miro, J. Pulsed Eddy Current Sensing for Critical Pipe Condition Assessment. Sensors. 17 (10), 2208 (2017).
Valls Miro, J., et al. A live test-bed for the advancement of condition assessment and failure prediction research on critical pipes. Proceedings of the Leading-Edge Strategic Asset Management Conference (LESAM13). , (2013).
Valls Miro, J., Ulapane, N., Shi, L., Hunt, D., Behrens, M. Robotic pipeline wall thickness evaluation for dense nondestructive testing inspection. Journal of Field Robotics. 35 (8), 1293-1310 (2018).
Valls Miro, J., Hunt, D., Ulapane, N., Behrens, M. Towards Automatic Robotic NDT Dense Mapping for Pipeline Integrity Inspection. Field and Service Robotics. , 319-333 (2018).
Chen, X., Lei, Y. Electrical conductivity measurement of ferromagnetic metallic materials using pulsed eddy current method. Non-Destructive Testing and Evaluation International. 75, 33-38 (2015).
Desjardins, D., Krause, T. W., Clapham, L. Transient eddy current method for the characterization of magnetic permeability and conductivity. Non-Destructive Testing and Evaluation International. 80, 65-70 (2016).
Chen, X., Lei, Y. Excitation current waveform for eddy current testing on the thickness of ferromagnetic plates. Non-Destructive Testing and Evaluation International. 66, 28-33 (2014).
Ulapane, N., Nguyen, L., Valls Miro, J., Alempijevic, A., Dissanayake, G. Designing a pulsed eddy current sensing set-up for cast iron thickness assessment. 12th IEEE Conference on Industrial Electronics and Applications (ICIEA). , 901-906 (2017).
Sophian, A., Tian, G., Fan, M. Pulsed eddy current non-destructive testing and evaluation: A review. Chinese Journal of Mechanical Engineering. 30 (3), 500 (2017).
Sophian, A., Tian, G. Y., Taylor, D., Rudlin, J. Design of a pulsed eddy current sensor for detection of defects in aircraft lap-joints. Sensors and Actuators A: Physical. 101 (1-2), 92-98 (2002).
Li, P., et al. System identification-based frequency domain feature extraction for defect detection and characterization. Non-Destructive Testing and Evaluation International. 98, 70-79 (2018).
Ulapane, N., Nguyen, L. Review of Pulsed-Eddy-Current Signal Feature-Extraction Methods for Conductive Ferromagnetic Material-Thickness Quantification. Electronics. 8 (5), 470 (2019).
Nguyen, L., Valls Miro, J., Shi, L., Vidal-Calleja, T. Gaussian Mixture Marginal Distributions for Modelling Remaining Pipe Wall Thickness of Critical Water Mains in Non-Destructive Evaluation. arXiv. , 01184 (2019).
Ulapane, N., et al. Gaussian process for interpreting pulsed eddy current signals for ferromagnetic pipe profiling. 2014 9th IEEE Conference on Industrial Electronics and Applications. , 1762-1767 (2014).
Ulapane, A. M. N. N. B. . Nondestructive evaluation of ferromagnetic critical water pipes using pulsed eddy current testing (Doctoral dissertation). , (2016).
Thiyagarajan, K., Kodagoda, S., Alvarez, J. K. An instrumentation system for smart monitoring of surface temperature. 2016 14thInternational Conference on Control, Automation, Robotics and Vision (ICARCV). , 1-6 (2016).
Thiyagarajan, K., Kodagoda, S., Van Nguyen, L. Predictive analytics for detecting sensor failure using autoregressive integrated moving average model. 2017 12th IEEE Conference on Industrial Electronics and Applications (ICIEA). , 1926-1931 (2017).
Thiyagarajan, K. . Robust sensor technologies combined with smart predictive analytics for hostile sewer infrastructures (Doctoral dissertation). , (2018).
Thiyagarajan, K., Kodagoda, S., Van Nguyen, L., Ranasinghe, R. Sensor failure detection and faulty data accommodation approach for instrumented wastewater infrastructures. IEEE Access. 6 (56), 562-574 (2018).
Thiyagarajan, K., Kodagoda, S., Ranasinghe, R., Vitanage, D., Iori, G. Robust sensing suite for measuring temporal dynamics of surface temperature in sewers. Scientific Reports. 8, 16020 (2018).
Thiyagarajan, K., Kodagoda, S., Van Nguyen, L., Wickramanayake, S. Gaussian Markov random fields for localizing reinforcing bars in concrete infrastructure. 35th International Symposium on Automation and Robotics in Construction. , 1052-1058 (2018).
Thiyagarajan, K., Kodagoda, S., Ulapane, N. Data-driven machine learning approach for predicting volumetric moisture content of concrete using resistance sensor measurements. 2016 IEEE 11th Conference on Industrial Electronics and Applications. , 1288-1293 (2016).
Giovanangelia, N., et al. Design and Development of Drill-Resistance Sensor Technology for Accurately Measuring Microbiologically Corroded Concrete Depths. 36th International Symposium on Automation and Robotics in Construction. , (2019).
Wickramanayake, S., Thiyagarajan, K., Kodagoda, S., Piyathilaka, L. Frequency Sweep Based Sensing Technology for Non-destructive Electrical Resistivity Measurement of Concrete. 36th International Symposium on Automation and Robotics in Construction. (771), (2019).
Ulapane, N., Wickramanayake, S., Kodagoda, S. Pulsed Eddy Current Sensing for Condition Assessment of Reinforced Concrete. 14th IEEE Conference on Industrial Electronics and Applications. , (2020).

Play Video

PDF

DOI

DOWNLOAD MATERIALS LIST

Citar este artículo

Ulapane, N., Thiyagarajan, K., Hunt, D., Valls Miro, J. Quantifying the Relative Thickness of Conductive Ferromagnetic Materials Using Detector Coil-Based Pulsed Eddy Current Sensors. J. Vis. Exp. (155), e59618, doi:10.3791/59618 (2020).