14.6:
Evenwichtsconcentratie Berekenen
40,539 Views
•
•
Being able to calculate equilibrium concentrations is essential to many areas of science and technology—for example, in the formulation and dosing of pharmaceutical products. After a drug is ingested or injected, it is typically involved in several chemical equilibria that affect its ultimate concentration in the body system of interest. Knowledge of the quantitative aspects of these equilibria is required to compute a dosage amount that will solicit the desired therapeutic effect.
A more challenging type of equilibrium calculation can be one in which equilibrium concentrations are derived from initial concentrations and an equilibrium constant. For these calculations, a four-step approach is typically useful:
- Identify the direction in which the reaction will proceed to reach equilibrium.
- Develop an ICE table.
- Calculate the concentration changes and, subsequently, the equilibrium concentrations.
- Confirm the calculated equilibrium concentrations.
Calculation of Equilibrium Concentrations
Under certain conditions, the equilibrium constant Kc for the decomposition of PCl5(g) into PCl3(g) and Cl2(g) is 0.0211. The above procedure can be used to determine the equilibrium concentrations of PCl5, PCl3, and Cl2 in a mixture that initially contained only PCl5 at a concentration of 1.00 M.
Step 1. Determine the direction the reaction proceeds.
The balanced equation for the decomposition of PCl5 is
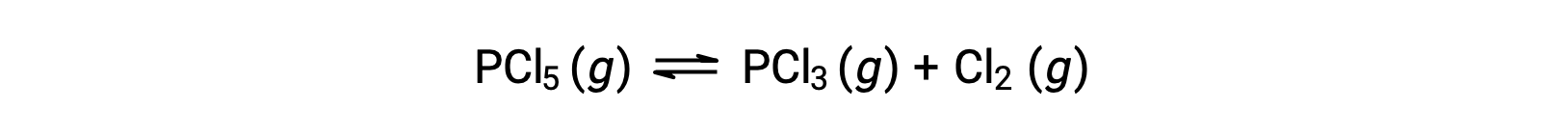
Because only the reactant is present initially, Qc = 0, and the reaction will proceed to the right.
Step 2. Develop an ICE table.
| PCl5 (g) | PCl3 (g) | Cl2 (g) | |
| Initial Concentration (M) | 1.00 | 0 | 0 |
| Change (M) | −x | +x | +x |
| Equilibrium Concentration (M) | 1.00 − x | x | x |
Step 3. Solve for the change and the equilibrium concentrations.
Substituting the equilibrium concentrations into the equilibrium constant equation gives
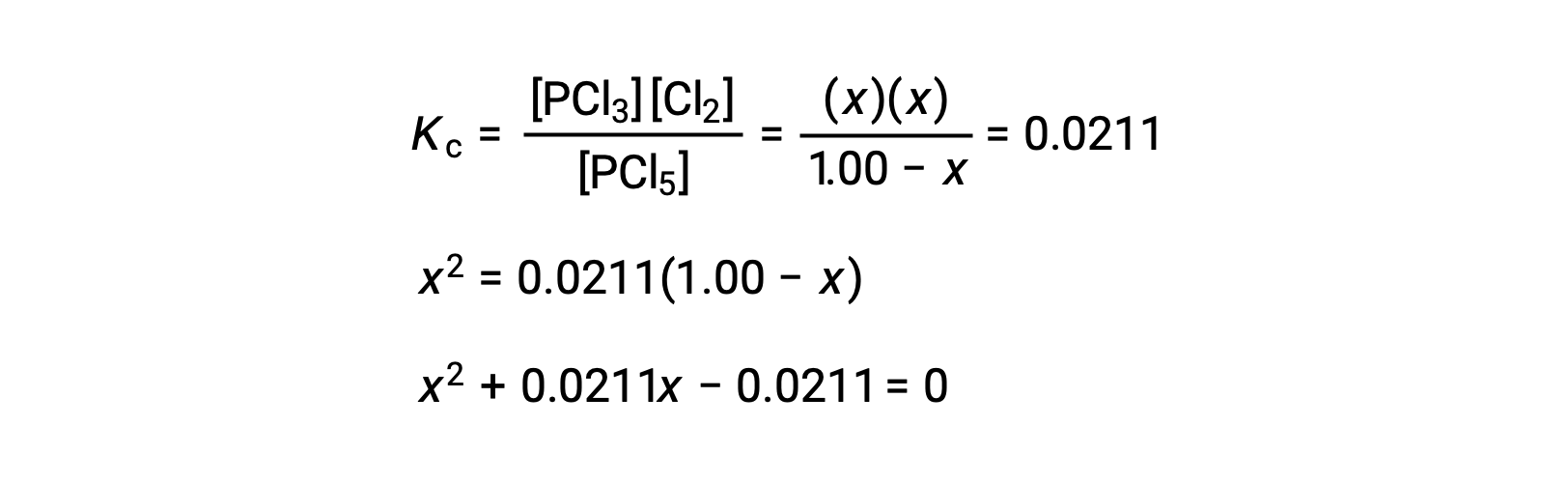
An equation of the form ax2 + bx + c = 0 can be rearranged to solve for x:
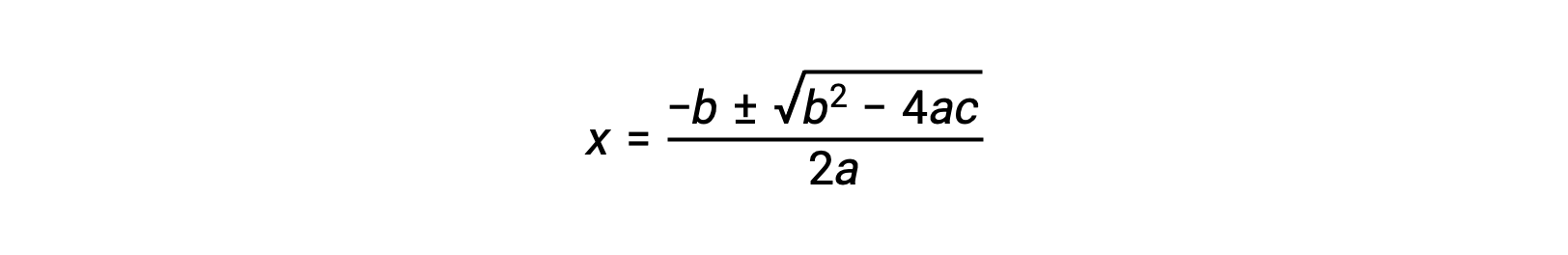
In this case, a = 1, b = 0.0211, and c = −0.0211. Substituting the appropriate values for a, b, and c yields:

The two roots of the quadratic are, therefore,
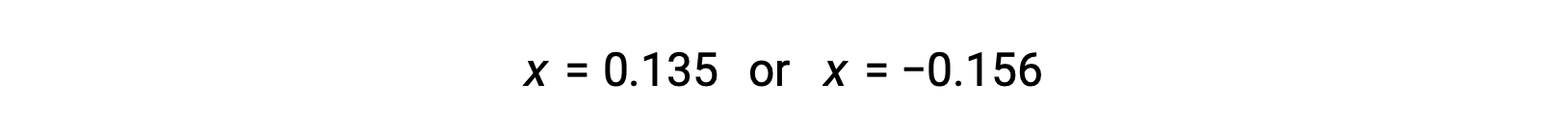
For this scenario, only the positive root is physically meaningful (concentrations are either zero or positive), and so x = 0.135 M. The equilibrium concentrations are
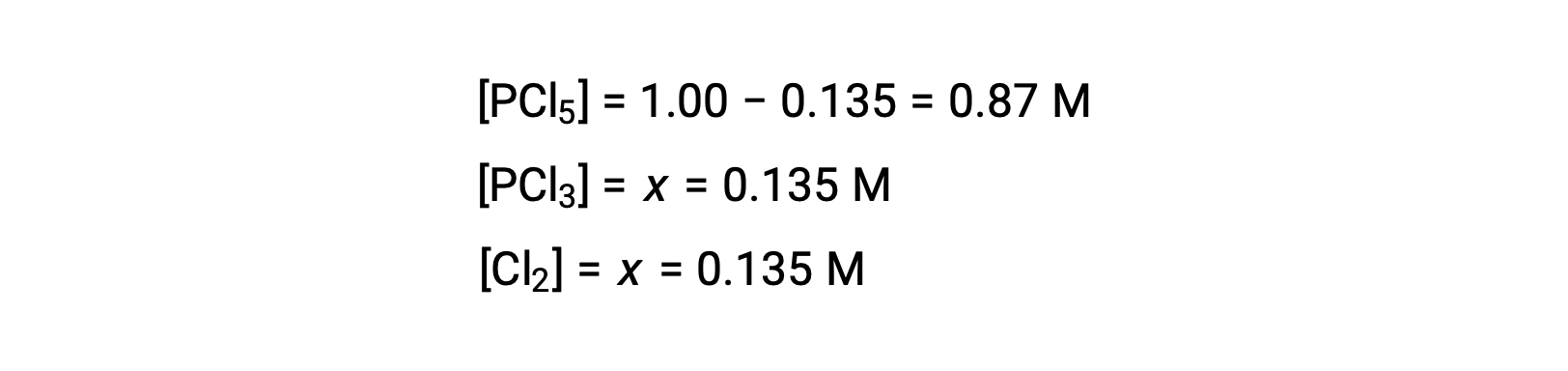
Step 4. Confirm the calculated equilibrium concentrations.
Substitution into the expression for Kc (to check the calculation) gives
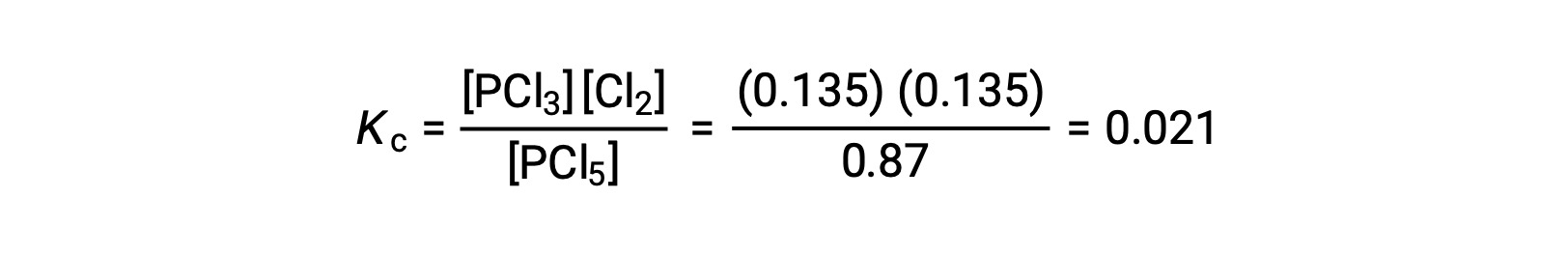
The equilibrium constant calculated from the equilibrium concentrations is equal to the value of Kc given in the problem (when rounded to the proper number of significant figures).
This text has been adapted from Openstax, Chemistry 2e, Section 13.4 Equilibrium Calculations.
