High Resolution Phonon-assisted Quasi-resonance Fluorescence Spectroscopy
Summary
The manuscript describes a method of phonon-assisted quasi-resonant fluorescence spectroscopy that incorporates both laser-limited resolution and photoluminescence (PL) spectroscopy. This method utilizes optical phonons to provide linewidth-limited resolution spectra of atom-like semiconductor structures in the energy domain. The method is also easily realized with a single spectrometer optical spectroscopy setup.
Abstract
High resolution optical spectroscopy methods are demanding in terms of either technology, equipment, complexity, time or a combination of these. Here we demonstrate an optical spectroscopy method that is capable of resolving spectral features beyond that of the spin fine structure and homogeneous linewidth of single quantum dots (QDs) using a standard, easy-to-use spectrometer setup. This method incorporates both laser and photoluminescence spectroscopy, combining the advantage of laser line-width limited resolution with multi-channel photoluminescence detection. Such a scheme allows for considerable improvement of resolution over that of a common single-stage spectrometer. The method uses phonons to assist in the measurement of the photoluminescence of a single quantum dot after resonant excitation of its ground state transition. The phonon's energy difference allows one to separate and filter out the laser light exciting the quantum dot. An advantageous feature of this method is its straight forward integration into standard spectroscopy setups, which are accessible to most researchers.
Introduction
High resolution is the key to unlocking new knowledge. With this knowledge, new technologies can be developed such as better sensors, more precise manufacturing tools, and more efficient computational devices. Generating this key, however, often comes at a high cost of resources, time or both. This issue is omnipresent across all scales from the atomic physics of resolving the lifted degeneracies of electron spins to astronomy where a small spectral shift can lead to detection of planets next to distant stars.1,2,3
The focus of this work is on using a standard spectrometer setup and showing how it can resolve spectral features below its resolution limit, especially with regard to the field of semiconductor optics. The example presented is that of anisotropic electron-hole (e-h) exchange splitting in InAs/GaAs quantum dots (QDs), which is on the order of a few µeV.4 The resolution limit of the spectrometer can be overcome by combining standard PL and laser spectroscopy techniques. This method of quasi-resonance fluorescence has the added benefit of achieving laser limited resolution using a commonplace single-stage spectrometer.
A standard optical spectroscopy system for single QD PL spectroscopy consists of a single-stage 0.3-0.75 m monochromator and a charge coupled device (CCD) detector along with an excitation laser source and optics. Such a system is at best capable of resolving 50 µeV in the near-infrared spectrum around 950 nm. Even with the use of statistical and deconvolution techniques, such a single monochromator setup is not capable of resolving less than 20 µeV in PL measurements.5 This resolution can also be improved by using a triple spectrometer, in triple additive mode, where the spectrum is successively dispersed by all three gratings. The triple spectrometer has the advantage of increased resolution, capable of resolving around 10 µeV. In an alternative configuration, triple subtractive mode, the first two gratings behave as a band pass filter, giving the added feature of being able to separate the excitation and detection by less than 0.5 meV. The drawback of the triple spectrometer is that it is a costly system.
Before presenting the method of interest, we briefly discuss other experimental approaches that, with added complexity, achieve better spectral resolution and are able to resolve the fine structure of single QDs. Elements of these methods are relevant to the presented method. One such method is adding a Fabry-Perot interferometer (FPI) in the detection path of a single spectrometer setup.6 Using this method the resolution is set by the finesse of the FPI. Thus, the spectrometer's resolution is improved to 1 µeV, at the cost of added complexity and lower signal intensity.7 The interferometer method also changes the general operation of the spectrometer with the CCD camera, effectively becoming a single point detector, and the tuning through various energies is achieved by adjusting the FPI cavity itself.
Resonance fluorescence (RF) spectroscopy, another method where a single optical transition is both excited and monitored also offers the promise of high-resolution spectroscopy. The spectral resolution is only limited by the laser linewidth and keeps the CCD as a multi-channel detector, where not just one sensor is detecting the signal but a number of CCD pixels. This multichannel detection is advantageous in terms of signal averaging. The challenge in RF spectroscopy is separating the PL signal from the larger background of the scattered laser light, especially when measuring at the single QD level. A number of techniques can be used to lower the ratio of signal to scattered laser light, which involve either polarization8, spatial9 or temporal separation10 of the excitation and detection. The first is to use high extinction polarizers to suppress the scattered light, but this method has the unfavorable outcome of losing polarization information from the PL.8 Another possible method to obtain resonance fluorescence is to engineer semiconductor systems that are coupled to optical cavities where the excitation and detection paths are spatially separated. This eliminates the issue of having to resolve the PL signal from the large laser background. However, this method is limited to intricate sample fabrication which is in general resource intensive.9
Another class of methods that is also able to resolve minute energy differences is that of pure laser spectroscopy, such as differential transmission, which has the benefit of achieving laser-limited resolution with complete polarization information. This method typically requires lock-in detection to observe miniscule changes in the transmission signal compared to that of the large laser background.11 Lately, advances in nanofabrication have led to a boost of the fraction of laser light that interacts with the QD(s) to values up to 20%, by either using index-matched solid immersion lenses or embedding the dots in photonic crystal waveguides.12
Even though these methods have the capability of achieving high energy resolution, they come at the cost of expensive equipment, complex sample fabrication and loss of information. The method in this work combines elements from these three methods without adding complexity in instrumentation or sample fabrication to a regular PL setup.
Recent work has shown that with a triple spectrometer system in subtractive mode, it is possible to visualize the singlet-triplet fine structure in the two-photon transition spectrum of a quantum dot molecule (QDM).13 The involved energy splitting on the order of a few to tens of μeV were resolved using a triple subtractive mode, which allowed to excite the transitions resonantly and detect within less than a meV. The spectral information was extracted by monitoring below the transition using acoustic phonons and other lower-lying exciton transitions. This method can also be applied to resolve the anisotropic e-h exchange splitting and even the lifetime-limited linewidth of the exciton transition of 8 µeV and 4 µeV, respectively as seen in Figure 1. Similar to this result, this paper will focus on a simple spectrometer setup that will incorporate many of the advantages that the other high resolution methods possess. Additionally the CCD will remain as a multi-channel detector. The experimental setup can also be kept fairly inexpensive relative to other high-resolution spectroscopy methods and has the added benefit of being easily modified to achieve single point correlation measurements. Unlike the result using acoustic phonons and a triple spectrometer, the underlying key is to make use of the LO-phonon satellite associated with the semiconductors and related alloys that make up semiconductor samples. The energy separation between LO-phonon satellite and the zero-phonon line (ZPL) is on the order of tens of meV for such samples, allowing the use of a single-stage spectrometer.14 This energy separation allows for use of the proposed quasi-resonance spectroscopy method by resonantly driving a transition and monitoring below the excitation by an energy equal to one LO phonon. This technique is analogous to that of PL excitation where one excites into an excited transition and monitors the ground state transition.15 The separation between the transition being excited and that of the LO-phonon satellite allows for the use of edge pass filters to suppress the elastically scattered light. This method of using the phonon satellite allows for laser linewidth limited resolution, since resonantly exciting the transition is typically the only time that the LO-phonon satellite emission becomes visible.
Protocol
Note: The methodology described is specific to a particular software, although other software packages may be used instead.
1. Sample Preparation and Cool Down
- Fabricate the sample.
- Grow the sample, using the Stranski-Krastanov growth method via molecular beam epitaxy creating two vertically-stacked self-assembled InAs/GaAs QDs that are separated by a 4 nm tunnel barrier as previously described.16 Embed the QDs in an electric field effect structure (i.e., Schottky diode) allowing for an electric field to be applied to the QDMs.17
Note: The use of QDMs is not a requirement for the method. Also, the semiconductors InAs/GaAs are not necessary, the technique will work for QDMs or QDs made from any semiconductor combination. - Fabricate the samples so that individual QDs can be optically addressed. Do this by either adding an aperture mask to the top of the sample or making a low density sample with 108 QDs/cm2 or less depending on the focal spot size.18
- Grow the sample, using the Stranski-Krastanov growth method via molecular beam epitaxy creating two vertically-stacked self-assembled InAs/GaAs QDs that are separated by a 4 nm tunnel barrier as previously described.16 Embed the QDs in an electric field effect structure (i.e., Schottky diode) allowing for an electric field to be applied to the QDMs.17
- Mount the sample into the chip header.
- Apply an alloy consisting of 50% bismuth, 26.7% lead, 13.3% tin, and 10% cadmium on the ceramic chip header. Heat the chip using a hot plate until the alloy liquefies. Place the bottom of the sample onto the liquefied alloy attaching it to the chip header.
Note: The bottom of the sample is one of the electrodes of the Schottky diode and the solder point to the chip header is connected to a pin. Another alternate for attaching the sample is conductive silver epoxy.
- Apply an alloy consisting of 50% bismuth, 26.7% lead, 13.3% tin, and 10% cadmium on the ceramic chip header. Heat the chip using a hot plate until the alloy liquefies. Place the bottom of the sample onto the liquefied alloy attaching it to the chip header.
- Adhere 40 G gold wire from a point (top corner) of the sample to a pin on the chip.
- Place a droplet of silver epoxy on the top corner of the sample and one droplet on one of the pin pads on the chip.
- Carefully lay the gold wire in the two droplets.
Note: The top of the sample is the other electrode of the Schottky diode that allows for application of an electric field.
- Mount the chip and sample into the cryostat and ensure the sample has good thermal contact with the copper sample holder.
- Apply indium foil between the chip and the cryostat's cold finger.
- Pressure mount the chip to the cold finger. Use two screws with washers and tighten firmly to ensure good thermal contact with the cryostat's copper cold finger.
- Attach wires from the chip pins that are connected to both the top and bottom electrodes on the sample. Run these wires through the cryostat to the source meter.
Note: The source meter applies a bias to the sample's electrodes, exposing the QDs to an external electric field. - Evacuate and bring the cryostat and sample chamber to vacuum. Start the turbo pump, evacuating to about 10-6 Torr in preparation for the cool down of the sample.
Note: Cooling and temperature control for the experiment is accomplished with the cryostat which is composed of a closed cycle refrigerator and an attached microscopy sample chamber. - Start the cryostat's compressor. Allow the cryostat to cool the system until reaching the desired temperature.
Note: For the presented results the temperature was approximately 18 K. Once the sample is cooled, the setup is ready for the installation of the optics that will allow for the optical measurement to be taken.
2. Optics Setup
Note: For all set up procedures, run the laser, source meter, spectrometer and CCD by either using the software provided by the manufacturer or other custom program.
- For PL collection, place a long working distance 50X microscope objective and collimation lens in line with the lens that focuses the PL signal onto the spectrometer. Collect the spectrum through a 0.75 m monochromator where the signal is dispersed by an 1,100 mm-1 grating and detected using a liquid nitrogen cooled 1,340 x 100 pixel CCD camera.
- Using a white light source, illuminate the sample.
- Focus the image of the sample through an external camera and the spectrometer by properly aligning the all optics on the detection side (i.e., collimation and focusing lens) and at zero wavelength get a clean focused image of the sample on the spectrometer CCD.
Note: It is helpful to have another external imaging camera to help with alignment and getting a crisp image of the sample.
- Focus the image of the sample through an external camera and the spectrometer by properly aligning the all optics on the detection side (i.e., collimation and focusing lens) and at zero wavelength get a clean focused image of the sample on the spectrometer CCD.
- After the detection side is set up, focus the laser onto the sample. Focus the beam spot to the smallest size possible on the sample by using a lens. Use a tunable diode laser with an excitation range that contains the ground state transition energies. Set the laser incident to the sample at an oblique angle. An advantage of oblique incidence is that it helps get rid of a large portion of the scattered laser light.
- Excite the sample at a higher non-resonant energy. Optimally, do this by exciting at an energy below the wetting layer. For the InAs/GaAs QDMs in this study this corresponds to 75 meV or more above the ground state transitions.
- Run the spectrum acquisition software in focus mode. Scan the sample across the laser spot using an X-Y translation stage which is attached to the cryostat microscopy sample housing. Do this until the spectrometer CCD captures the discrete lines of the ground-state transitions. Center the detection on one of the QDMs.
Note: Once a QDM is found, the optical setup is completed. For the samples used the ground state energies are around 1,300 meV. - Generate a bias map.
- Apply a potential via the source meter (step 1.5) connected to the electrodes on the sample; this applies a bias across the electrodes in turn generating an electric field to the QDMs.
Note: The bias range applied to the sample is 0-2 V across the Schottky diode structure. This is when the device is in reverse bias, and the electric field limits the amount of charges in the QDM allowing for the individual charge states to be visible. - Take individual spectra at different incremented voltage values, depending on desired resolution this normally ranges from hundredths to thousandths of a volt. Combine these individual spectra together by using a custom program (e.g., LabView).
Note: The program can be easily coded using a wide range of different programs to combine the vector columns of the individual spectrums into a matrix, in the presented experiment this has been added in to stitch together the data in real-time.- Click the run button to take a bias map. This takes spectra at a set bias and make it a column vector, then adds each incremented bias spectra as another column.
Note: This generates a data matrix where the intensity values correspond to the PL intensity, rows represents energy/wavelength, and columns correspond to voltage. The bias map should be viewable as it is being run, thus allowing live feedback on the quality of the data.
Note: A bias map helps to identify different charge configurations and gives the proper information to complete the setup of both the excitation and detection paths.
- Click the run button to take a bias map. This takes spectra at a set bias and make it a column vector, then adds each incremented bias spectra as another column.
- Apply a potential via the source meter (step 1.5) connected to the electrodes on the sample; this applies a bias across the electrodes in turn generating an electric field to the QDMs.
- Identify the transition that will be excited. Note the energy of the transition and the bias range of interest.
- At this point decide how the laser excitation will be tuned through the transition. There are three different options to get the laser to excite into the ground state transition:
- Tune the transition energy by temperature.18
- Use the Stark shift of the transition to achieve resonance with the laser energy.19
Note: A nice feature of the two methods mentioned above is that a tunable laser source is not needed, since the QDM transitions are tuned through a fixed laser energy. - Alternatively, use a tunable laser source, stepping the laser energies through the transition. A detection signal will be present when the laser is resonant with the transition, this gives the measurement its laser limited spectral resolution. This will be the focus for the rest of the protocol.
- At this point decide how the laser excitation will be tuned through the transition. There are three different options to get the laser to excite into the ground state transition:
- With the transition identified and the experimental parameters set, select both excitation and detection energies for the measurement.
- Choose the excitation energy as that of the transition. Choose the detection as the excited transition's energy minus the energy of the longitudinal optical (LO) phonon associated with the semiconductor alloy. Using these values, choose the proper edge pass filters for the measurement; they must have cutoffs in between the excitation and detection energies.
Note: For the experimental results presented, the driven transition was the neutral ground state exciton shown in Figure 3, observed at 1,301.7 meV and the -1 LO phonon emission is located at 1,266 meV, corresponding to 952.5 nm and 979.3 nm, respectively. We therefore use a 960 nm short pass filter for the excitation and a 960 nm long pass filter for the detection. Interference cut-off filters are ideal for this purpose since they can be tuned by adjusting the angle.
- Choose the excitation energy as that of the transition. Choose the detection as the excited transition's energy minus the energy of the longitudinal optical (LO) phonon associated with the semiconductor alloy. Using these values, choose the proper edge pass filters for the measurement; they must have cutoffs in between the excitation and detection energies.
- Set the laser to excite at the transition energy of interest, which is done simply by entering the desired laser wavelength value in the appropriate input field on the front panel of the laser control software.
- Set the center wavelength to the predetermined value to monitor the -1 LO photon emission by entering the desired center wavelength value in the appropriate input field on the front panel of the spectrometer control software.
Note: The -1 LO photon emission for InAs/GaAs is approximately 36 meV below the exciton transition that will be excited. - Using the camera software, start collection with the CCD by running the spectrum acquisition software in continuous mode by clicking the focus mode button. A signal should be visible or could still be hidden by the laser scatter.
- Maximize the signal. CRITICAL STEP: Tune the excitation short pass filter, by slightly adjusting its angle so that it has the proper wavelength cutoff.
Note: The optimal angle is established by monitoring the signal while adjusting the angle of the short pass filter. By changing the angle of the filter this changes the cut-off wavelength. The key is to make sure that as much laser light as possible is suppressed from the collection.
3. Quasi-resonance Measurement Setup
- Set experimental parameters on the computer controls using the custom software’s main screen. To do so, start the collection program and click on the Step Polarization, Temp, or WL tab. This sets all the experimental values and once run, collects data through the various parameters.
Note: For our experiment’s data taking all computer controls were custom programmed. The key is to have a software or program able to set the center wavelength of the spectrometer, control the CCD and source meter, and step the laser energy through the transition while collecting a range of spectrums at different biases.- Input the established laser energy range that the laser will scan through: from about 50 µeV above the neutral exciton transition energy of 1,301.7 meV, to 50 µeV below. Set the initial Wavelength to start the scan using the “Desired WL (nm)” field. Set the end range of the laser to scan over (“End Motor Unit”).
- Set the bias range that the source meter will scan through by clicking on the “Voltage Settings” tab. Set the starting bias value (“Voltage Start (V)”), the end bias value “Voltage End (V)” and the bias step size “Voltage Step (V)”. Here, the bias range scanned was 1.68 to 1.82 V.
- Input the integration time chosen by clicking on the “Camera Settings” tab. Set the integration time for the CCD under “Exposure (s)” (Refer to step 3.3). Select a reasonable integration time for the CCD. Experiment with the integration time to get a good signal. The larger the integration time the better signal averaging that is obtainable.20
Note: Integration times used for the experiment were 10 sec. But, integration times can be as low as 0.5 sec, depending on the strength of the PL signal. At times it is not even necessary to see the signal in individual scans but upon stitching the spectra together the data summation plus the capability of the eye to interpolate will reveal the transitions in the photoluminescence excitation (PLE) map.
4. Data Acquisition
- Once the experimental parameters are established begin the experiment. Start collection by clicking the run button.
Note: At each laser energy the software varies the bias taking a spectrum and a background spectrum. This is done for each bias step. Then the laser energy is varied and the process continues until the entire range chosen is complete. - Post-process the data.
- Take the additional background scans that are taken at the end of every bias map and subtract the average from each of the bias columns. Use a background subtraction program, or write a program that takes the background columns, averages them together and subtract this averaged background spectra from each bias column in the data matrix. Note: See Supplemental Code File for the program used in our lab.
Note: This removes other spurious signals caused by any remaining scattered laser light, greatly improving the bias map.
- Take the additional background scans that are taken at the end of every bias map and subtract the average from each of the bias columns. Use a background subtraction program, or write a program that takes the background columns, averages them together and subtract this averaged background spectra from each bias column in the data matrix. Note: See Supplemental Code File for the program used in our lab.
- Analyze the data.
- For example, to extract the characteristic parameters of the spectral line use a mathematical fitting software to run a Lorentzian fitting at each bias slice of the PL map.21 Once the fitting procedure has been completed all information will be in the coefficients of the fitting such as the maximum intensity, spectral location and FWHM.
Representative Results
The results presented in the figures show the high resolution capabilities of using phonons to assist in the PL measurement. The schematic (Figure 2) shows that, with the exception of the edge pass filters on both excitation and detection, the experimental setup remains a standard spectroscopy setup, with the optional addition of polarization control. Comparison with a single and triple spectrometer (Figure. 3) portrays the phonon-assisted method's great improvement to resolution. The anisotropic e-h splitting is clearly displayed allowing for accurate measurements of the splitting (Figure 4). The method also allows one to easily make lifetime-limited linewidth measurements of QD transitions (Figure 5). Fitting the peaks with Lorentzian functions completes analysis of the data; extrapolating from the fits, it is possible to extract both the splitting and full width half maximum. Furthermore, this quasi-resonance technique can be incorporated with a triple spectrometer in triple subtractive mode (Figure 1) to monitor transitions within 0.5 meV.
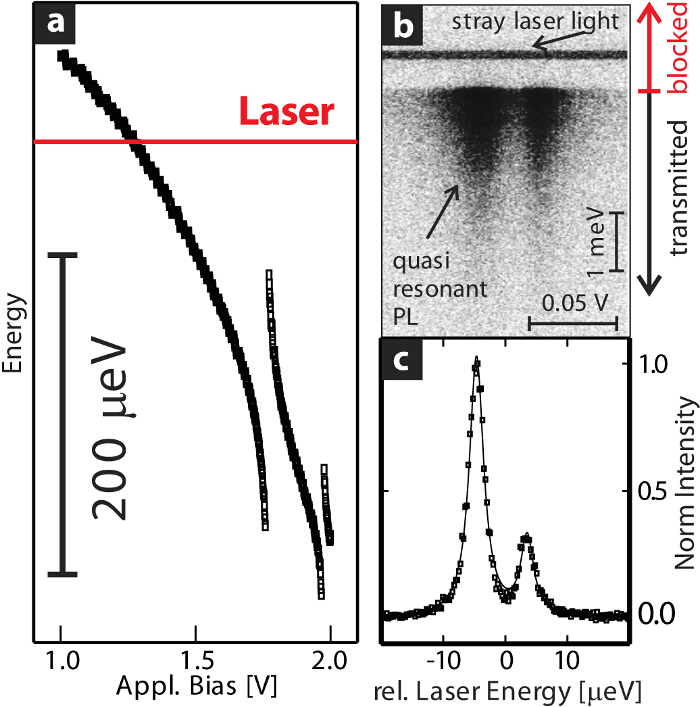
Figure 1. Acoustic-Phonon-Assisted Measurement. Capabilities of the quasi-resonance spectroscopy technique. (A) Peak intensity of the ground state neutral exciton of a QDM as seen in PL. The red line indicates the quasi-resonance excitation. (B) PL in the tail of the exciton transition as the transition is tuned into resonance with the laser. Using a triple spectrometer in triple subtractive mode, the excitation and detection are separated by less than 1 meV. (C) Summed quasi-resonant PL from (B), depicting resolution of features of the anisotropic e-h exchange splitting and the lifetime-limited linewidth of the transition. Please click here to view a larger version of this figure.

Figure 2. Experimental Setup Schematic. Schematic representation of the simple spectrometer setup that is used for the LO-phonon-assisted measurements. Indicated are the tunable diode laser, both long-pass (LP) and short-pass (SP) filters used for tuning the region of detection, the microscope objective (MO), the spectrometer, and liquid nitrogen cooled CCD. The dashed boxes on both the excitation and detection represent the optional components of a variable retarder (VR) and polarizers (Pol) necessary for polarization measurements. Please click here to view a larger version of this figure.
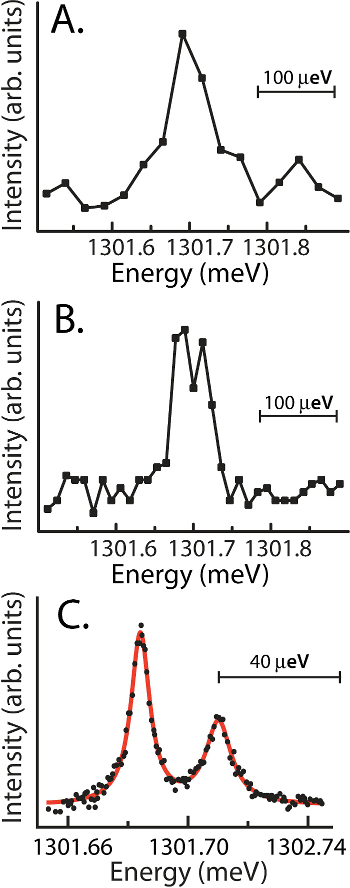
Figure 3. Spectral Resolution Comparison of Three PL-based Methods. Example of the achievable resolution using different methods; in A and B, the spectrometer gratings and the CCD pixel width limit the resolution. (A) Neutral exciton transition as resolved by a single spectrometer with non-resonant excitation around 918 nm. The spectral resolution is about 26 µeV per pixel and is too large to be able to make out the anisotropic e-h exchange splitting. (B) The same spectral region as in (A) with non-resonant excitation, but with the spectrometer set in triple additive mode, where the resolution is 10 µeV. (C) Neutral exciton transition as resolved by using the phonon satellite in this quasi-resonant phonon-assisted spectroscopy method. The two peaks are well resolved and fit by a double Lorentzian function, which yields an anisotropic e-h exchange splitting of 23.3 ± 0.1 µeV. The extracted FWHM values for lower and higher energy peaks are 7.3 ± 0.1 µeV and 9.6 ± 0.4 µeV, respectively. Please click here to view a larger version of this figure.
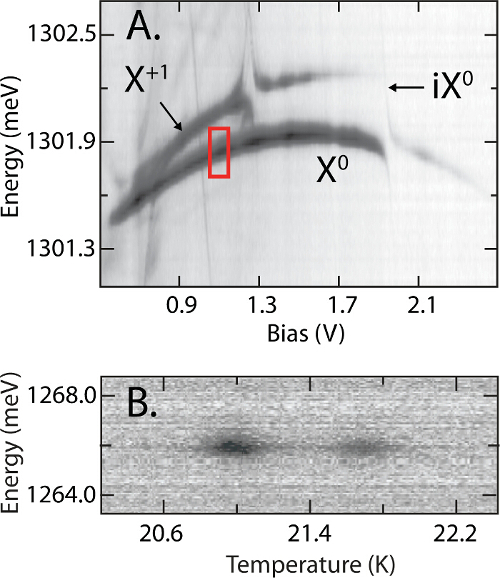
Figure 4. PL Map of a QDM and Associated Phonon-assisted Measurement. (A) Regular resolution bias map of the QDM under non-resonant excitation. The bias map shows emission from the neutral direct (X0) and indirect (iX0) exciton, as well as the positive trion (X+). Also, the bias at which the laser is scanned through is indicated by the red box at around 1.1 V. (B) High resolution PL at -1 phonon satellite below the excitation through the direct neutral exciton. The transition energy was tuned through a fixed laser energy of 951.657 nm (1,302.824 meV) by stepping the temperature. The -1 phonon satellite is seen to be about 36 meV below the zero phonon lines. Please click here to view a larger version of this figure.

Figure 5. Bias Map of Anisotropic e-h Splitting. Bias map of the anisotropic e-h exchange splitting, centered at 1,302.28 meV. The bias map was made by incrementing the voltage applied by 2 mV increments at each laser energy and stepping the laser energy 37 times across the energy range, roughly changing about 1.7 µeV in each step. The average of the e-h exchange energy is 25.4 µeV with a standard deviation of 0.8 µeV over this bias region. The fitting of the Stark shift is displayed. Please click here to view a larger version of this figure.
Discussion
The above instructions demonstrate the phonon-assisted quasi-resonance spectroscopy method. By exciting into a QD discrete state, one can monitor the phonon emission line, achieving high resolutions. In the example provided, by using phonons it is even possible to resolve the lifetime-limited linewidth of the neutral exciton visible in experiments. The method is easy to incorporate into existing PL spectroscopy setups. As mentioned, once the energy of the desired transition line is identified via non-resonant spectroscopy, the center wavelength of the spectrometer is established by setting it -1 LO phonon below that transition. This energy difference is used to make the measurement by employing short pass and long pass filters on the excitation and detection path, respectively. The filters are necessary to suppress any side modes of the laser light that might reach the spectrometer due to the relatively close proximity of both the excitation and detection energies. It is this reduction of stray laser light that is a crucial element that maximizes the detected signal.
By tuning the laser through the transition energy, the -1 phonon satellite will emit when the laser is in exact resonance with the desired transition; this is due to the -1 LO phonon being a higher-order process. This crucial feature of the measurements is what allows for the experimental system to have laser limited resolution. Scanning the laser through the transition can be completed in two different ways, the first of which is using a tunable laser source. Using laser tuning the resolution is set by the step size by which the laser can be varied. This step size must be less than the width of the energy structures to be resolved. Alternatively, a second method is to keep the laser energy fixed and scan the transition energy. In most systems this can be achieved in two different ways: first, by changing the sample temperature, which tunes the band gap as described by the Varshni law and consequently sweeps the transition energy through the excitation;22 second, by applying an electric field, which Stark shifts the exciton transition energy and allows tuning of the transition through the laser energy.23 A useful feature of the Stark shift method is higher spectral resolution for smaller Stark shifts; this is due to the laser-limited nature of the measurement.
This optical spectroscopy method has many favorable properties. It is a convenient method of investigating the anisotropic e-h exchange splitting energy, lifetime-limited linewidth and has the potential to resolve even smaller energy differences. Another favorable aspect of this experimental technique is that full polarization information can be accessed. In the presented example of a coupled QD, the neutral exciton ground state has a bright doublet that is distinguished by its spin configuration. The degeneracy of this doublet is lifted in QDs due to strain and shape anisotropies. Electron-hole anisotropies for these samples range from near zero up to tens of µeV. If both branches of the e-h exchange splitting doublet are excited, polarization information in the photoluminescence can be obtained by utilizing a polarization analyzer, consisting of a polarizer and variable retarder in the detection path. This is due to the phonons not carrying any spin information, thus the complete polarization information of the spectra would be contained in the optical emission. On the other hand, using this setup allows for selective polarization excitation by having the liquid crystal retarder rotate the polarization on the excitation side to coincide with individual branches of the splitting. Polarization control is achieved by adding both a variable retarder and linear polarizer in both the excitation and detection paths. This allows for excitation and detection of select polarizations, giving both absorption and emission polarization information about the sample.
The main limitation of this high resolution measurement method is the restriction to materials that support optical phonons, such as semiconductors. For such a measurement to be completed, the material must have an optical phonon energy associated with it, since this energy difference enables performing the measurement with a standard single spectrometer. This method allows for taking high resolution spectra of quantum dots and other zero-dimensional systems. For example, the method can just as well be applied to study atomic defects in materials, such as nitrogen vacancy centers in diamond and defects in silicon carbide.24, 25 While the method works great for low-dimensional semiconductor structures, it is not necessarily of benefit to the study of other structures or even material, as spectral broadening may eliminate the need for high resolution or coupling to LO-phonons may be too weak. Aside from these limitations the measurement technique holds several advantages over the other methods mentioned in the manuscript.
This paper has demonstrated a highly functional resonant fluorescence spectroscopy method, providing laser limited resolution with standard PL gathered by a single stage spectrometer by using material properties of the -1 optical phonon satellite. This method is a powerful tool with many uses in resolving energy features that are less than 10 µeV while retaining polarization information in the collected spectra, as demonstrated by resolving the anisotropic e-h exchange splitting of QDs.
Offenlegungen
The authors have nothing to disclose.
Acknowledgements
The authors would like to acknowledge Allan Bracker and Daniel Gammon at the Naval Research Laboratory for providing the samples being studied. This work was supported (in part) by the Defense Threat Reduction Agency, Basic Research Award # HDTRA1-15-1-0011, to University of California-Merced.
Materials
| Tunable Diode Laser DL pro | Toptica Photonics | DL Pro | |
| Closed Cycle Cryogen Free Refrigerator System for Microscopy | Cryo Industries of America Inc. | Cryocool G2 | |
| Sourcemeter | Keithley | 2611a | |
| 50x Mitutoyo Plan Apo NIR Infinity-Corrected Objective | Mitutoyo America Corporation | 378-825-5 | |
| Turbo pump | Pfeiffer Vacuum | HiPace 80 | |
| NIR coated Mirrors | Thor labs | BB1-E03 | |
| Polarizers | Thorlabs | LPNIR050-MP | |
| 200mm AR coated Achromatic lens | Thorlabs | AC254-200-B-ML | |
| 100mm AR coated Achromatic lens | Thorlabs | AC254-100-B-ML | |
| 960 Long pass filter | Thorlabs | 960aelp | |
| 960 Short pass filter | Thorlabs | 960aesp | |
| Liquid Crystal Variable Retarder | Meadowlark Optics | LVR-100 | |
| 0.75m Spectrometer Acton SpectraPro | Princeton Instruments | Trivista | |
| Liquid Nitrogen Cooled Camera | Princeton Instruments | 7508-0002 | |
| External Camera | Watec | Wat-902H Ultimate | Optional |
| Ostoalloy | Lake Shore Cryotronics | Ostalloy 158 | |
| Gold wire (40 gauge) | Surepure Chemetals | Au-Wire-03-02 | |
| Silver Epoxy | A.I. Technology | Prima-Solder EG8020 | |
| Program Software | National Instruments | LabView |
Referenzen
- Germanis, S., et al. Piezoelectric InAs/GaAs quantum dots with reduced fine-structure splitting for the generation of entangled photons. Phys. Rev. B. 86, 1-4 (2012).
- Valenti, J. A., Fischer, D. A. Spectroscopic Properties of Cool Stars (SPOCS). I. 1040 F, G, and K Dwarfs from Keck, Lick, and AAT Planet Search Programs. ApJ. 159, 141-166 (2005).
- Oetiker, B., et al. Searching for Companions to Late Type M Stars. .Astro. Soc. Pac. Conf. Ser. 212, (2000).
- Seguin, R., Rodt, S., Schliwa, A., Potschke, K., Pohl, U. W., Bimberg, D. Size-dependence of anisotropic exchange interaction in InAs/GaAs quantum dots. Phys. Status Solidi B. 243 (15), 3937-3941 (2006).
- Belhadj, T., et al. Controlling the Polarization Eigenstate of a Quantum Dot Exciton with Light. Phys. Rev. Lett. 103 (1-4), (2009).
- Ulrich, S. M., et al. Control of single quantum dot emission characteristics and fine structure by lateral electric fields. Phys. Status Solidi B. 246 (2), 302-306 (2009).
- Vamivakas, A. N., et al. Observation of spin-dependent quantum jumps via quantum dot resonance fluorescence. Nature. 467, 297-300 (2010).
- Poem, E., et al. Polarization sensitive spectroscopy of charged quantum dots. Phys. Rev. B. 76, (2007).
- Flagg, E. B., et al. Resonantly driven coherent oscillations in a solid-state quantum emitter. Nature Phys. 5, 203-207 (2009).
- Scheibner, M., Bacher, G., Forchel, A., Passow, T., Hommel, D. Spin Dynamics in CdSe/ZnSe Quantum Dots: Resonant vesus Nonresonant Excitation. J. Supercond. Nov. Magn. 16 (2), 395-398 (2003).
- Faelt, S., Atature, M., Tureci, H. E., Zhao, Y., Badolato, A., Imamoglu, A. Strong electron-hole exchange in coherently coupled quantum dots. Phys. Rev. Lett. 100, 1-4 (2008).
- Vamivakas, A. N., et al. Strong Extinction of a Far-Field Laser Beam by a Single Quantum Dot. Nano Letters. 7 (9), 2892-2896 (2007).
- Scheibner, M., Economou, S., Ponomarev, I. V., Jennings, C., Bracker, A., Gammon, D. Two-Photon Absorption by a Quantum Dot Pair. Phys. Rev. B. 92, (2015).
- Palik, E. D. . Handbook of Optical Constants of Solids. Vols. I and II. , (1985).
- Kerfoot, M. L., et al. Optophononics with Coupled Quantum Dots. Nat. Commun. 5, 1-6 (2013).
- Scheibner, M., Bracker, A. S., Kim, D., Gammon, D. Essential concepts in the optical properties of quantum dot molecules. Solid State Commun. 149, 1427-1435 (2009).
- Bracker, A. S. Engineering electron and hole tunneling with asymmetric InAs quantum dot molecules. Appl. Phys. Lett. 89, 1-3 (2006).
- Doty, M. F., et al. Electrically Tunable g Factors in Quantum Dot Molecular Spin States. Phys. Rev. Lett. 97, 1-4 (2006).
- Stinaff, E. A., et al. Optical Signatures of Coupled Quantum Dots. Science. 311, 636-639 (2006).
- Tkachenko, N. V. . Optical Spectroscopy: Methods and Instrumentations. , (2006).
- Hecht, E. . Optics. , (2014).
- O’Donnell, K. P., Chen, X. Temperature dependence of semiconductor band gaps. Appl. Phys. Lett. 58, 2924-2926 (1991).
- Stinaff, E. A., et al. Polarization dependent photoluminescence of charged quantum dot molecules. Phys. Stat. Sol. (c). 5 (7), 2464-2468 (2008).
- Jelezko, F., Wrachtrup, J. Single defect centres in diamond: A review. Phys. Stat. Sol. (a). 203 (13), 3207-3225 (2006).
- Doherty, M. W. The nitrogen-vacancy colour centre in diamond. Physics Reports. 528 (1), 1-45 (2013).

